Introduction to Geometry

Content

This course provides an overview of the evolution of the field of Geometry from Ancient Greece to the twentieth century. This is done on the basis of the Erlangen programme, an overarching vision developed by the mathematician Felix Klein that links group theory and geometry. In particular, the following geometries are considered: Euclidean Geometry, Affine Geometry, Projective Geometry, and Hyperbolic Geometry. It is briefly discussed which objects are studied by those geometries, which techniques they use and which symmetries are important. We also discuss the nature of conic sections in these different geometries and tie this vision to other courses in geometry such as Algebraic and Differential geometry.

Topics

  • Euclidean Geometry
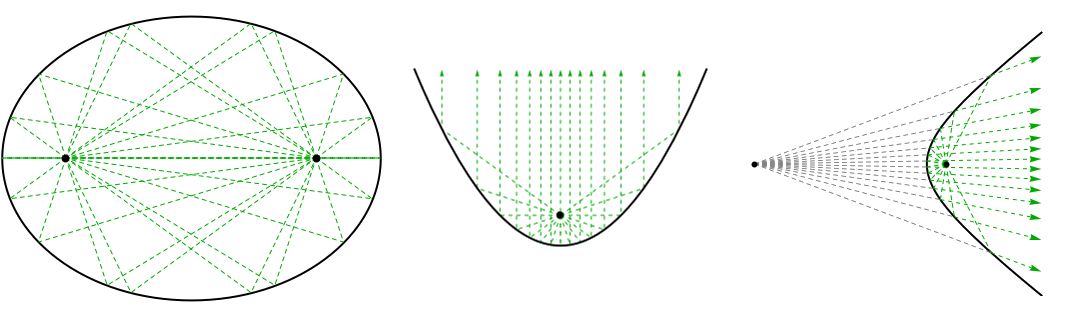
  • Conic Sections
  • Affine transformations
  • The projective plane
  • Projective transformations
  • Hyperbolic geometry
  • The Erlangen Program

Study Material

  • Literature
    Literature: Brannan et al., 'Geometry'.

  • Syllabus
    A fill-in syllabus is available

Learning goals

  • Students can describe the Erlangen program and illustrate it with examples.
  • Students can describe the basic concepts from the following geometries: Euclidean Geometry, Affine Geometry, Projective Geometry, Hyperbolic Geometry, etc.
  • Students can recognize from which geometry a particular theorem comes.
  • Students can use techniques from different types of geometry to prove geometric theorems.
  • Students can describe the symmetry groups of the following geometries: Euclidean Geometry, Projective Geometry, Hyperbolic Geometry, Differential Geometry and Algebraic Geometry.
  • Students can describe and apply the classification of conic sections in the different geometries.
  • Students can provide a historical overview of the evolution of Geometry from Antiquity to the present day.